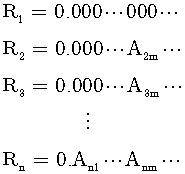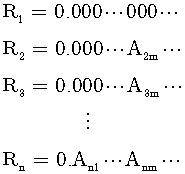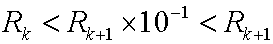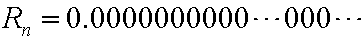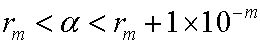Is the Infinite Decimal Available?
We cannot write an infinite decimal completely. Anyone has not seen it. Further, Kevin Delaney casts doubt on the validity of it in Repeating Nines. Nonetheless, a real number is represented by it in the modern mathematics. Especially, Cantor's diagonal argument uses a list of infinite decimals. After Cantor, mathematicians accept actual infinity. The infinite decimal is a concept of actual infinity. Before Cantor, mathematicians rejected actual infinity. They accepted only potential infinity. The infinite decimal does not exist in potential infinity. Let us consider whether the infinite decimal is available or not.
In "What Is Infinity?", I say that an arbitrary natural number is finite. The proof by mathematical induction is below.
- 1 is a finite number.
- Assume that n is a finite number, then n+1 is a finite number.
- Therefore, an arbitrary natural number is finite.
In potential infinity, no matter how many digits a decimal has, it is a finite decimal. For example, we shall consider the decimal expansion of π. We can approximate π by the decimal expansion but some error remains. Obviously, it is a finite decimal and a rational number. Furthermore, the value of the decimal expansion of π is not fixed. It approaches π without limit, but the value of it changes every time we compute one more digit.
In actual infinity, the infinite decimal expansion of π is an irrational number and fixed. Because it absolutely equals π, we cannot remove or add a digit. Furthermore, there is a possibility to calculate π to an arbitrary number of digits but it is impossible to reach infinity. The reason is that the number of digits is a natural number, and any natural number is finite, according to the above mathematical induction. Inevitably, we say that the infinite decimal expansion of π is only in the idea world. It remains stationary. However, the decimal expansion of π is not fixed in potential infinity. How does it change into the infinite form. How is its infinite form fixed in the idea world? These questions are difficult to answer.
Next, we shall consider strange examples. The strangeness is caused by the infinite decimal. The critical problem is as follows. Mathematical induction cannot be applied to the infinite decimal, because it is a concept of actual infinity. However, each digit of it is determined by computation. This fact means that we can apply mathematical induction to every digit of it.
Consider all real numbers in the interval [0,1] in actual infinity. They are represented by infinite decimals. We shall try to arrange them in ascending order. Because every real number has a infinite decimal representation, the second smallest positive real number has it. Of course, there are infinite decimal representations of the third and fourth and so on. The resulting list is represented below:
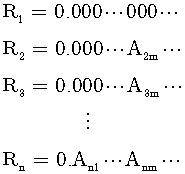 We represent the n-th smallest number of the list as Rn and the m-th digit of Rn as Anm. Let us consider step by step. We are going to determine each digit of the list:
We represent the n-th smallest number of the list as Rn and the m-th digit of Rn as Anm. Let us consider step by step. We are going to determine each digit of the list:
- Assume that R1 is 0. Then, all digits of R1 equal 0.
- Assume that Akm is zero. Then, A(k+1)m is zero. This is proved by contradiction. If A(k+1)m weren't zero, then Rk+1X10-1 would be smaller than Rk+1 and lager than Rk:
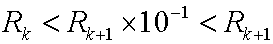 This contradicts the premise that Rk+1 is (k+1)-th smallest number in the interval [0,1].
This contradicts the premise that Rk+1 is (k+1)-th smallest number in the interval [0,1].
- According to the mathematical induction, an arbitrary digit of the list is zero.
That is, an arbitrary digit of Rn is zero.
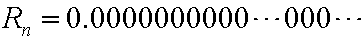 Then, we cannot determine the value of Rn. This strange situation is caused by the infinite decimal.
Then, we cannot determine the value of Rn. This strange situation is caused by the infinite decimal.
Next, Consider all real numbers in the interval [0,π] in actual infinity. We shall try to arrange them in descending order. Then, we can use the same logic as the above list. The resulting list is represented below:
 These real numbers correspond to only one Dedekind's cut. That is, the Dedekind's cut contains infinite irrational numbers. This contradicts Dedekind's definition of the real number. That is, the infinite decimal is the paradoxical concept.
These real numbers correspond to only one Dedekind's cut. That is, the Dedekind's cut contains infinite irrational numbers. This contradicts Dedekind's definition of the real number. That is, the infinite decimal is the paradoxical concept.
We shall use only finite decimals to avoid paradoxes. Let us consider all m-digit decimals in the interval [0,1]. Now, the second smallest number is obviously 1X10-1. An arbitrary irrational number α can be approximated by the m-digit decimal rm:
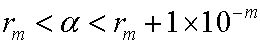 If we need more accuracy, we can use more digits. So I think that the finite decimal is enough available. Furthermore, John Gabriel says that there is no need to interpret 0.999... as being equal to 1. There is no inevitability to use the infinite decimal. Irrational numbers are represented by other forms: Therefore, I think that infinite decimals are not necessary.
If we need more accuracy, we can use more digits. So I think that the finite decimal is enough available. Furthermore, John Gabriel says that there is no need to interpret 0.999... as being equal to 1. There is no inevitability to use the infinite decimal. Irrational numbers are represented by other forms: Therefore, I think that infinite decimals are not necessary.
Table of Contents